Source: Phys.org

Disclaimer: Copyright infringement not intended.

Context

• A discovery led by researchers from the Max Planck Institute in Marburg and Philipps University in Marburg has unveiled the first regular molecular fractal found in nature.

Details

• This discovery challenges previous notions about molecular structures and sheds light on the intricate complexities of biological evolution.
• The study, published in Nature, highlights the accidental emergence of a microbial enzyme, citrate synthase from a cyanobacterium, that forms a pattern known as the Sierpinski triangle—a remarkable example of self-similarity at the molecular level.

Background

• Fractals, characterized by repeating patterns across scales, are rare in nature but are found in various structures such as snowflakes, fern leaves, and romanesco cauliflower heads.
• While molecules exhibit a degree of regularity, they typically appear smooth at a distance, lacking the self-similarity observed in fractals.

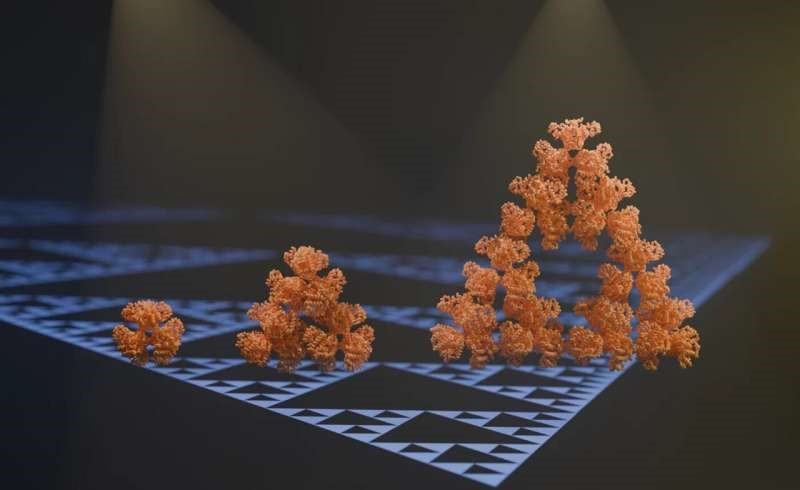
What are Fractals?

• Fractals are complex geometric shapes that exhibit self-similarity at different scales.
• This means that when you zoom into a fractal, you'll see smaller copies of the overall shape, each with similar patterns to the whole.
• Fractals often possess infinite detail, meaning you can continue to zoom in and discover new patterns, albeit at diminishing scales.

Properties of Fractals:

• Self-Similarity: Fractals exhibit self-similarity, meaning that parts of the fractal resemble the whole at different scales.
• Fine Structure: Fractals possess intricate, detailed structures at all scales, with complexity increasing as you zoom in.
• Non-Integer Dimension: Unlike regular geometric shapes, fractals can have non-integer dimensions, such as fractional or fractal dimensions.

Types of Fractals:

• Deterministic Fractals: These fractals are generated using mathematical formulas or algorithms, such as the Mandelbrot set or the Koch snowflake.
• Random Fractals: Also known as stochastic fractals, these are generated using randomness or statistical processes, such as fractal landscapes or Brownian motion.

Applications of Fractals:

• Computer Graphics: Fractals are widely used in computer graphics to generate realistic natural phenomena like mountains, clouds, and foliage.
• Data Compression: Fractal compression algorithms exploit the self-similarity of images to achieve high compression ratios.
• Fractal Antennas: These antennas utilize fractal geometry to achieve compact size and multiband performance in wireless communication systems.
• Finance: Fractal patterns are observed in financial markets, with techniques like fractal analysis used for market prediction and risk assessment.
• Biological Modeling: Fractals are employed to model and understand complex structures in biology, such as branching patterns of blood vessels and lung airways.
• Art and Design: Many artists and designers use fractals as inspiration for creating visually stunning artworks and patterns.

Sources:

Phys.org

PRACTICE QUESTION Q.  Fractals represent a intersection of mathematics, art, science, and technology. Examine. (250 Words)